Trigonometry on the unit circle
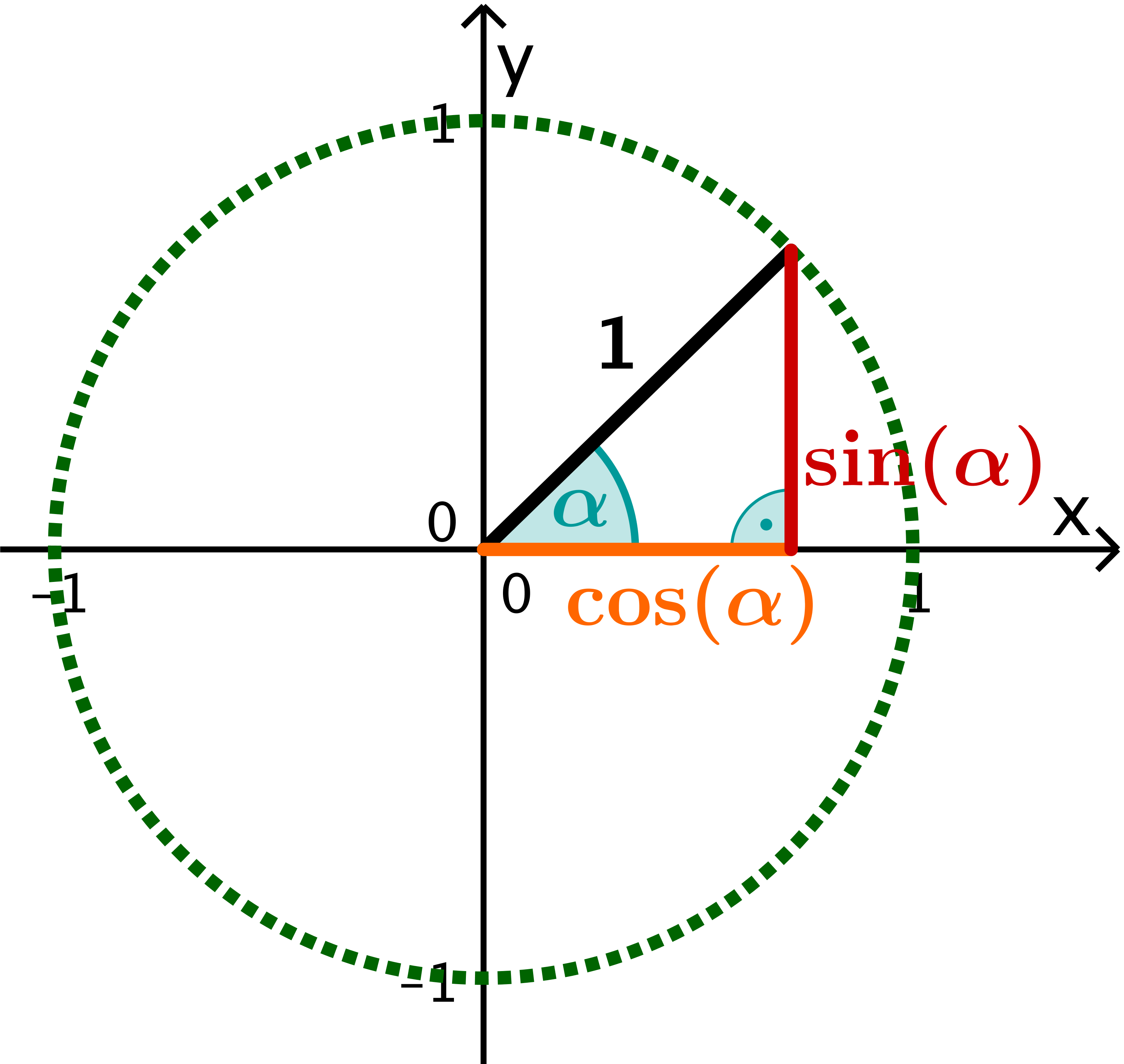
If you apply the angle to the -axis, you can read off the values of the sine and cosine of on the unit circle.
If you look at the right triangle with angle in the unit circle, the hypotenuse has length :
Presign
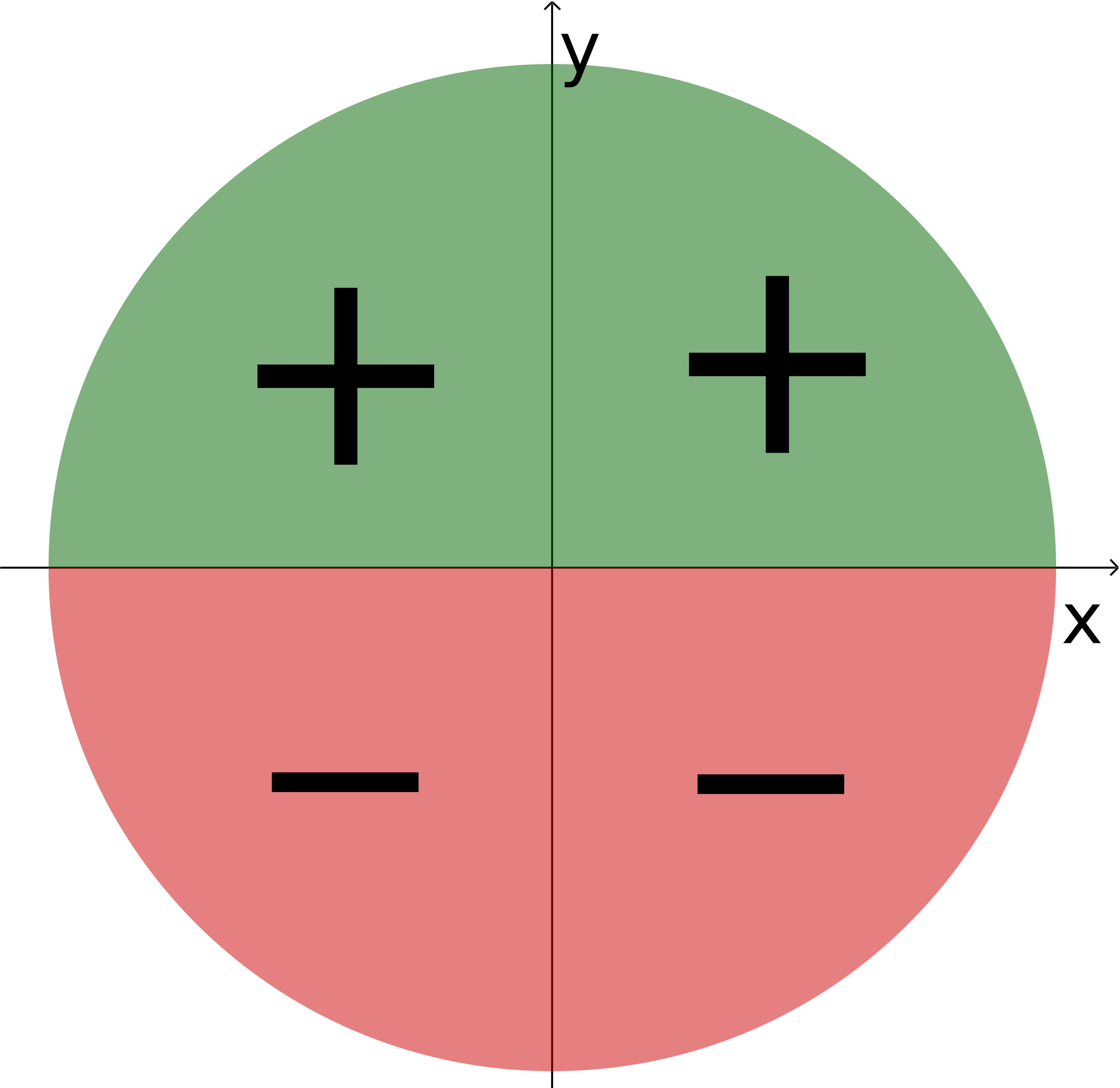
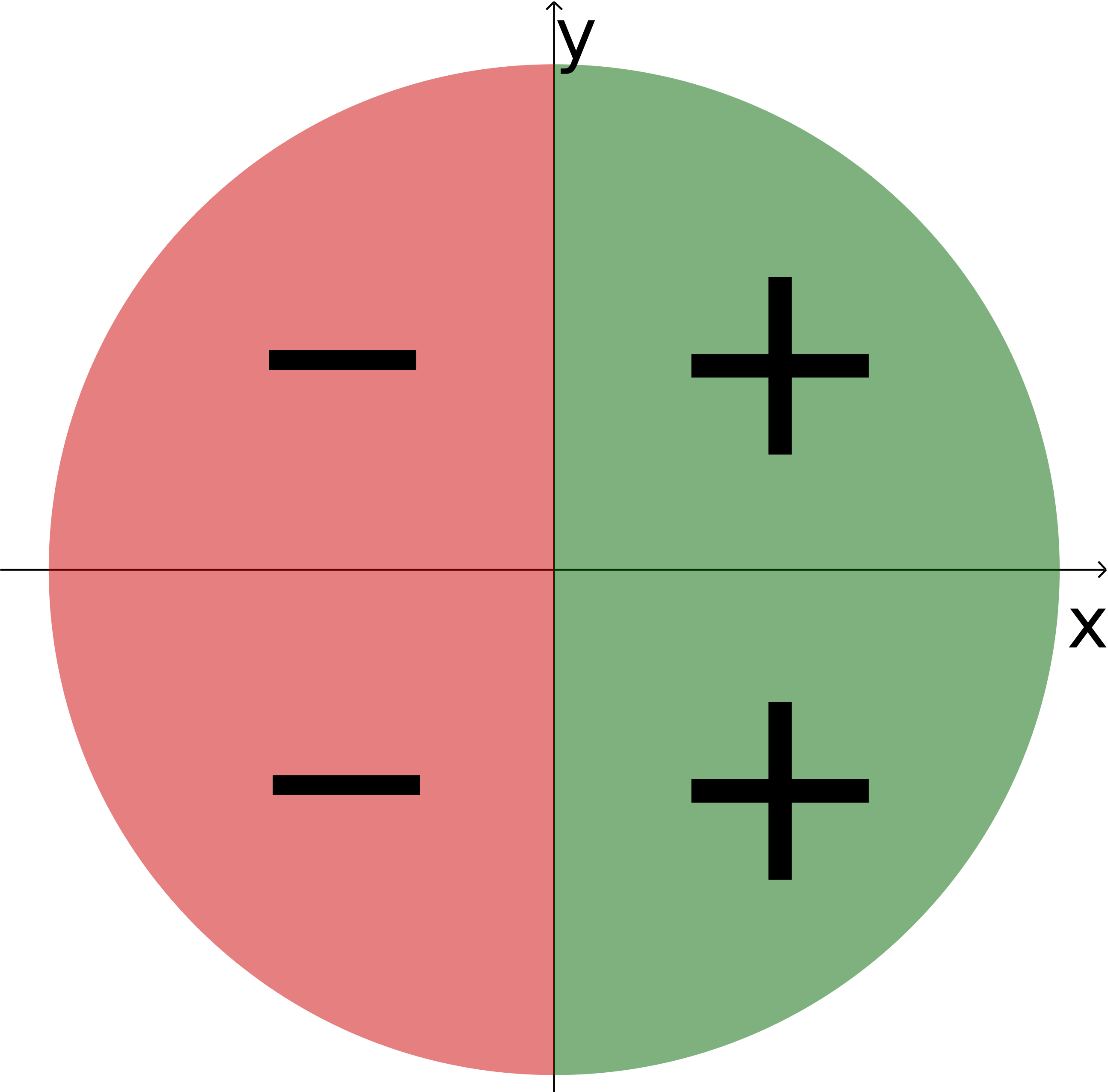
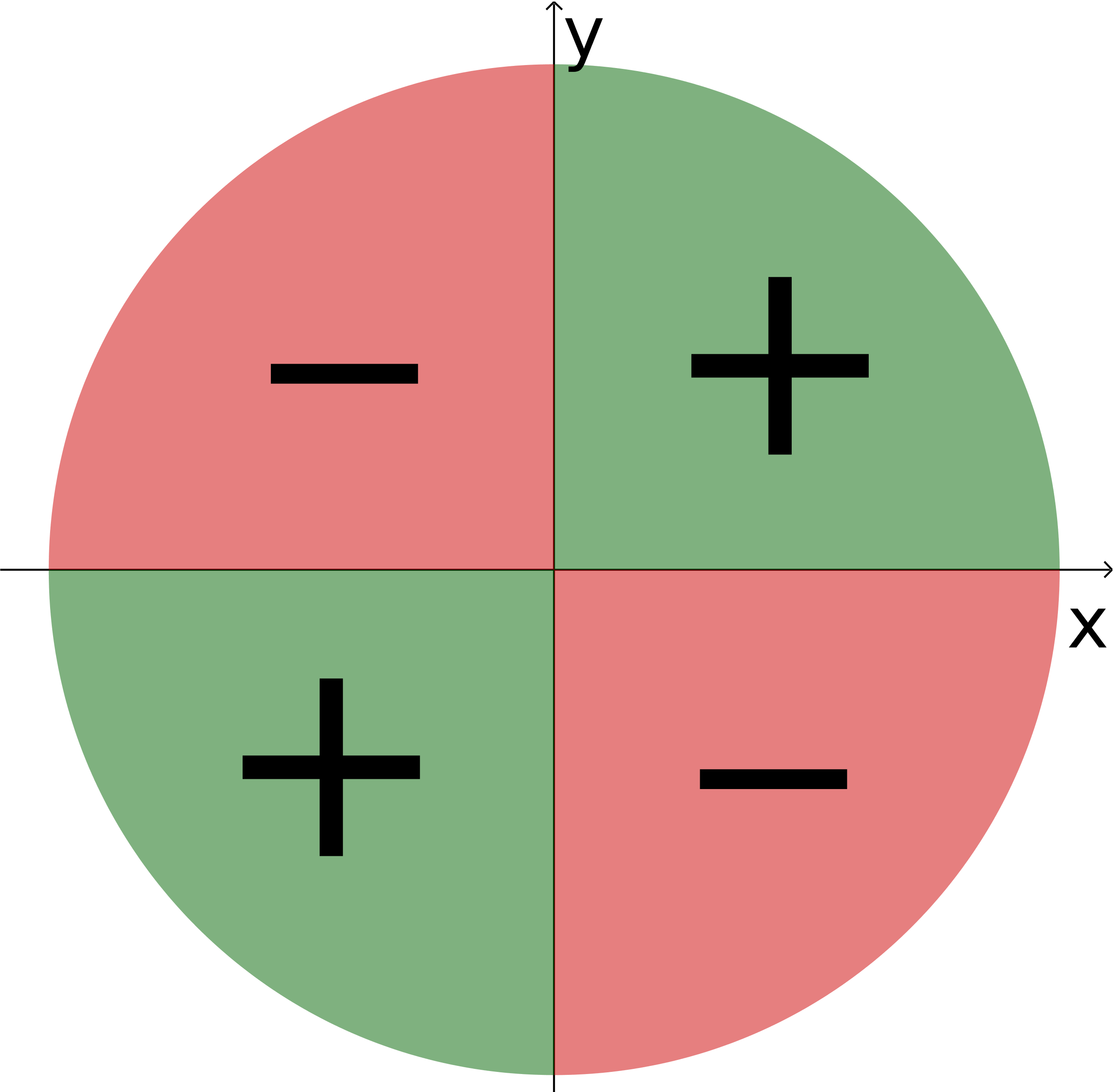
The trigonometric functions can change their sign when passing from one quadrant to the next. The change is illustrated in the following graphs.
Sine
Cosine
Tangent
Important values
The table below lists some important values for the trigonometric functions. For the first five values of the sine and cosine, there is an easy way to remember the values. They have the general form , where you put the numbers to in ascending order for the sine and in descending order for the cosine.
Angle | ||||||||
|---|---|---|---|---|---|---|---|---|
: At these points the tangent does not exist, because the tangent is defined as "sine over cosine" and at these points the cosine is equal to zero.
Visualization by an applet
Video about the tangent on the unit circle
Exercises
Loading