ln-function

The logarithm function with base $e$ , i.e., Euler's number, is called the natural logarithm or $\ln$ -function. It is specified by:

f : ℝ^{+} \to ℝ, x \mapsto \ln (x)

Here, $\ln (x)$ is the logarithm with base $e$ , that is, $\ln (x) = \log_{e} (x)$ .

Eigenschaften

Die $\ln$ -Funktion hat die gleichen Eigenschaften wie Logarithmusfunktionen zu beliebigen Basen. Weil $e \approx 2,718 > 1$ ist sie monoton steigend.

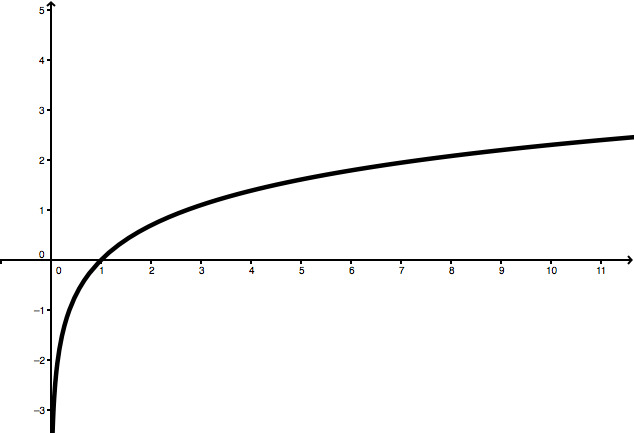
Graph der $\ln$ -Funktion:

Beziehung zu anderen Funktionen

Umkehrfunktion

Die Umkehrfunktion der $\ln$ -Funktion ist die $e$ -Funktion. Für $f (x) = \ln (x)$ gilt also:

f^{- 1} (x) = e^{x} \Rightarrow e^{\ln (x)} = x = \ln (e^{x})

Ableitung

Die Ableitung von $f (x) = \ln (x)$ , ist gegeben durch:

f^{'} (x) = \frac{1}{x}

Stammfunktion

Das erste Integral bzw. eine Stammfunktion zu $f (x) = \ln (x)$ lautet:

F (x) = x \cdot \ln (x) - x

Zur Herleitung bzw. Berechnung der Stammfunktion siehe den Artikel Partielle Integration.

Beliebige Logarithmusfunktion als ln-Funktion

Einen Logarithmus $l o g_{a} (x)$ zu einer beliebigen Basis $a$ (mit $a \in ℝ^{+}$ , $a \neq 1$ ), kannst du über folgende Formel in eine ln-Funktion überführen:

\log_{a} (x) = \frac{\ln (x)}{\ln (a)}

Exercises

You can find more exercises in the following folder::
Gemischte Aufgaben zur e- und ln-Funktion

Still want more?

You can find more content on this topic here:

Articles

This content is licensed under
CC BY-SA 4.0 → What does that mean? serlo.org