Exercises: Sine, Cosine and Tangent on a right triangle
- 1
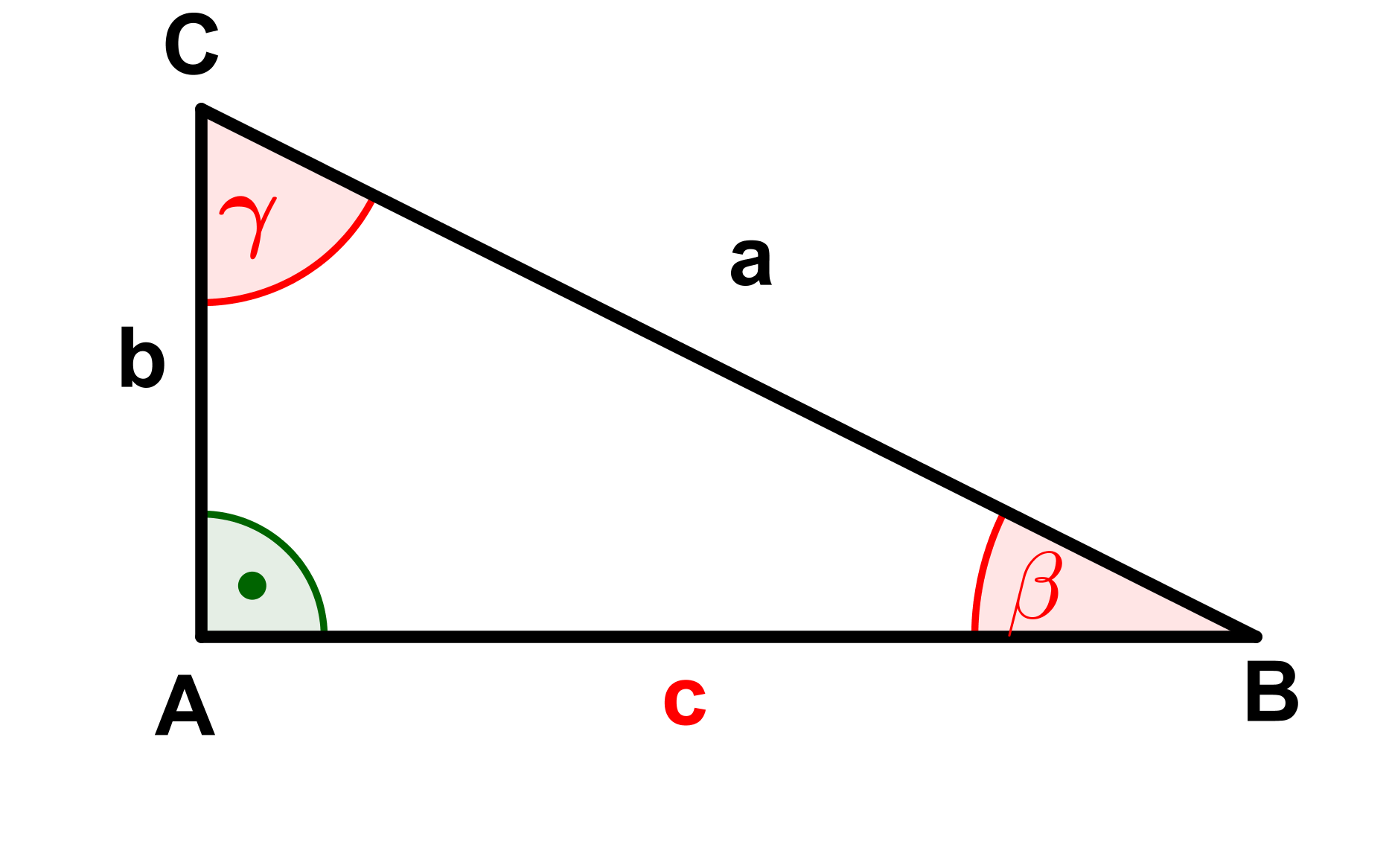
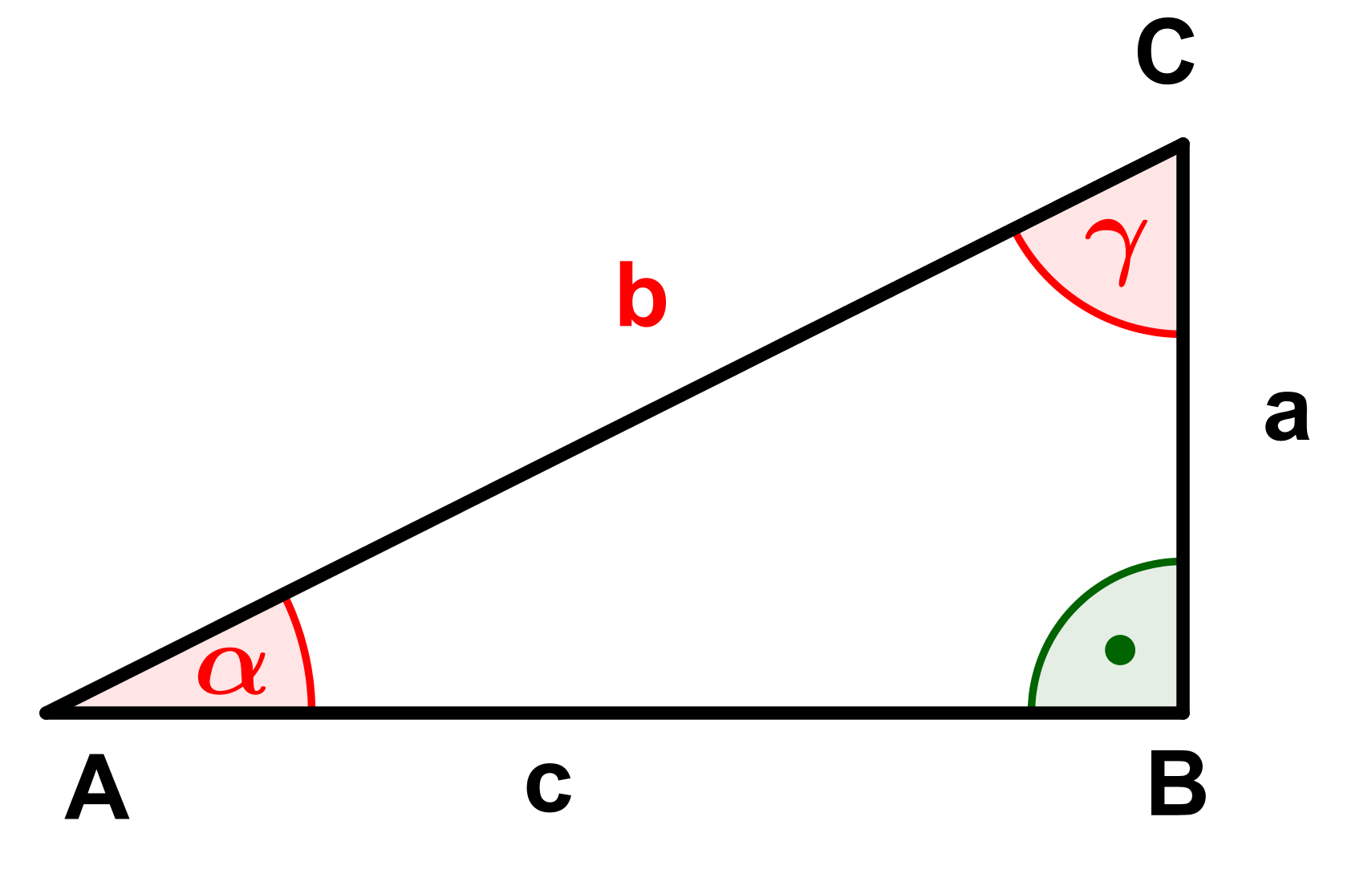
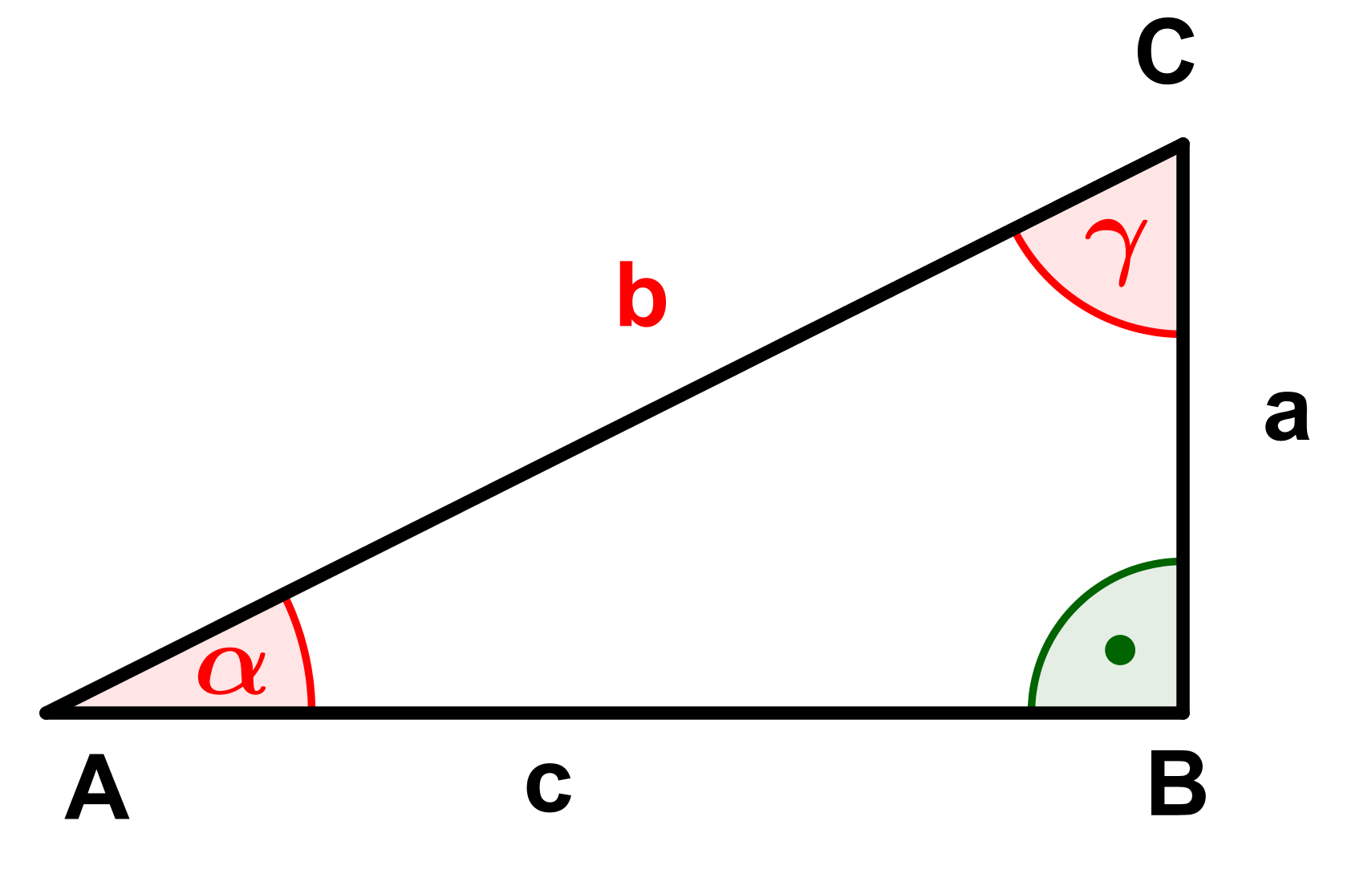
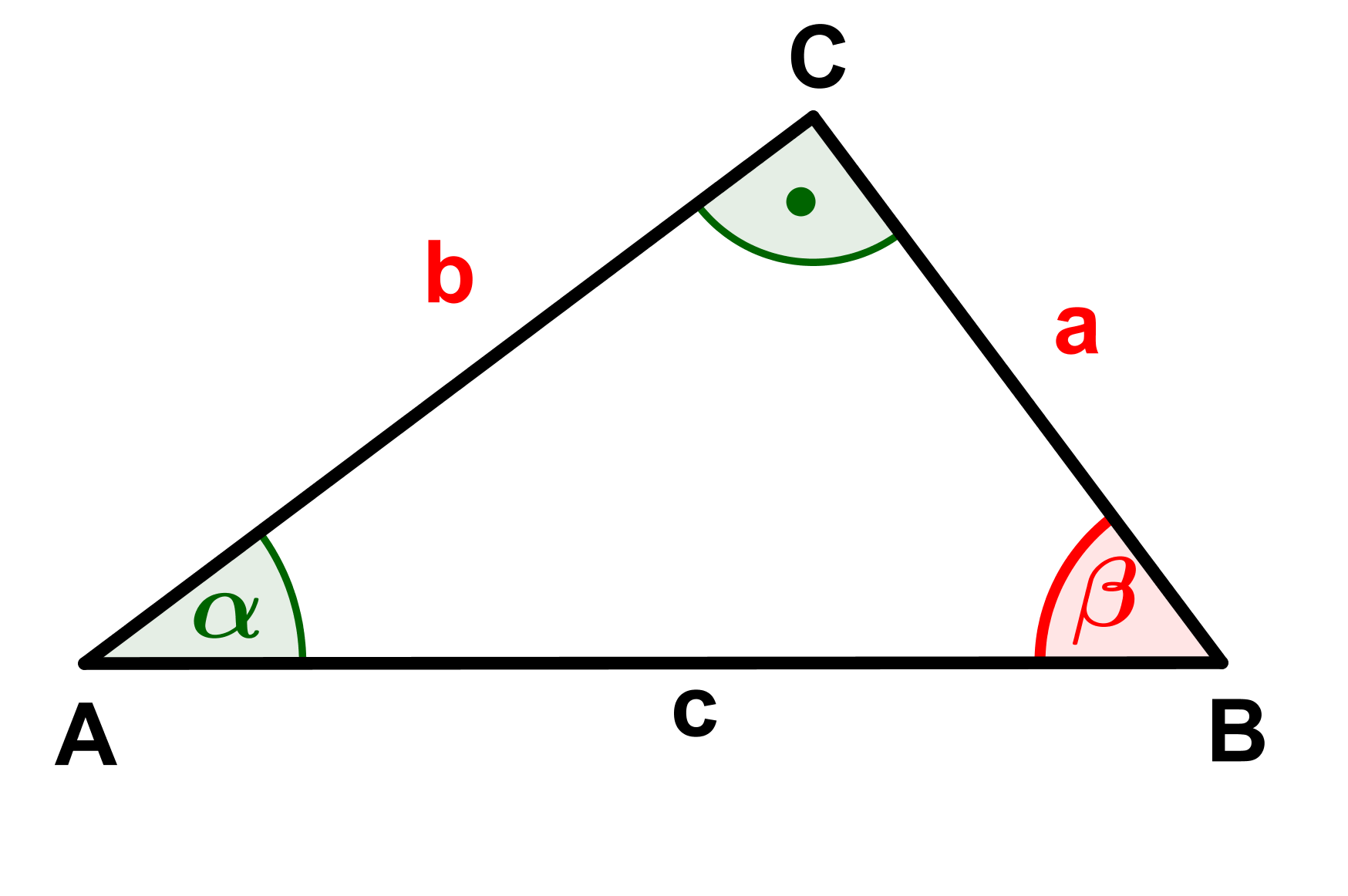
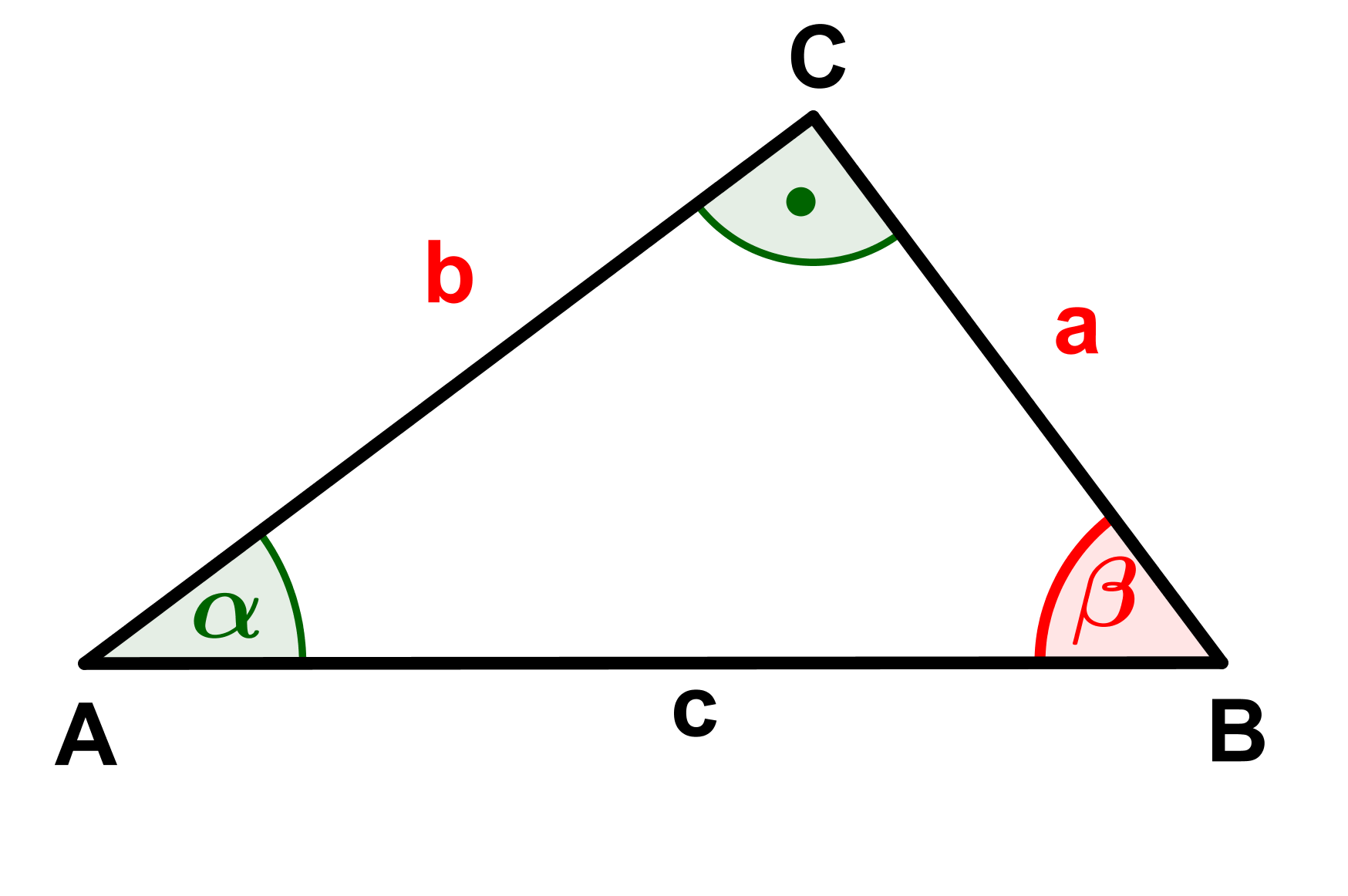
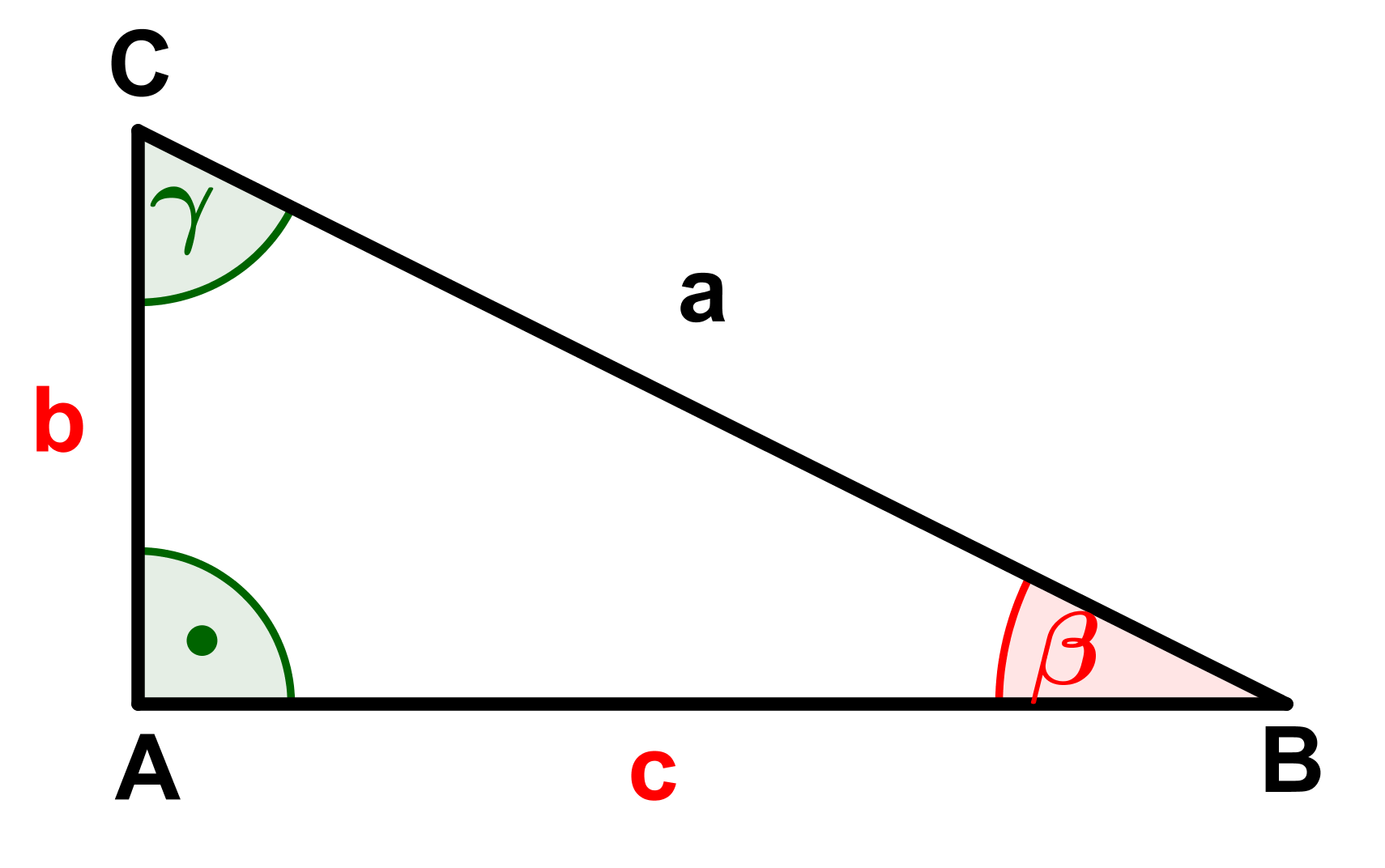
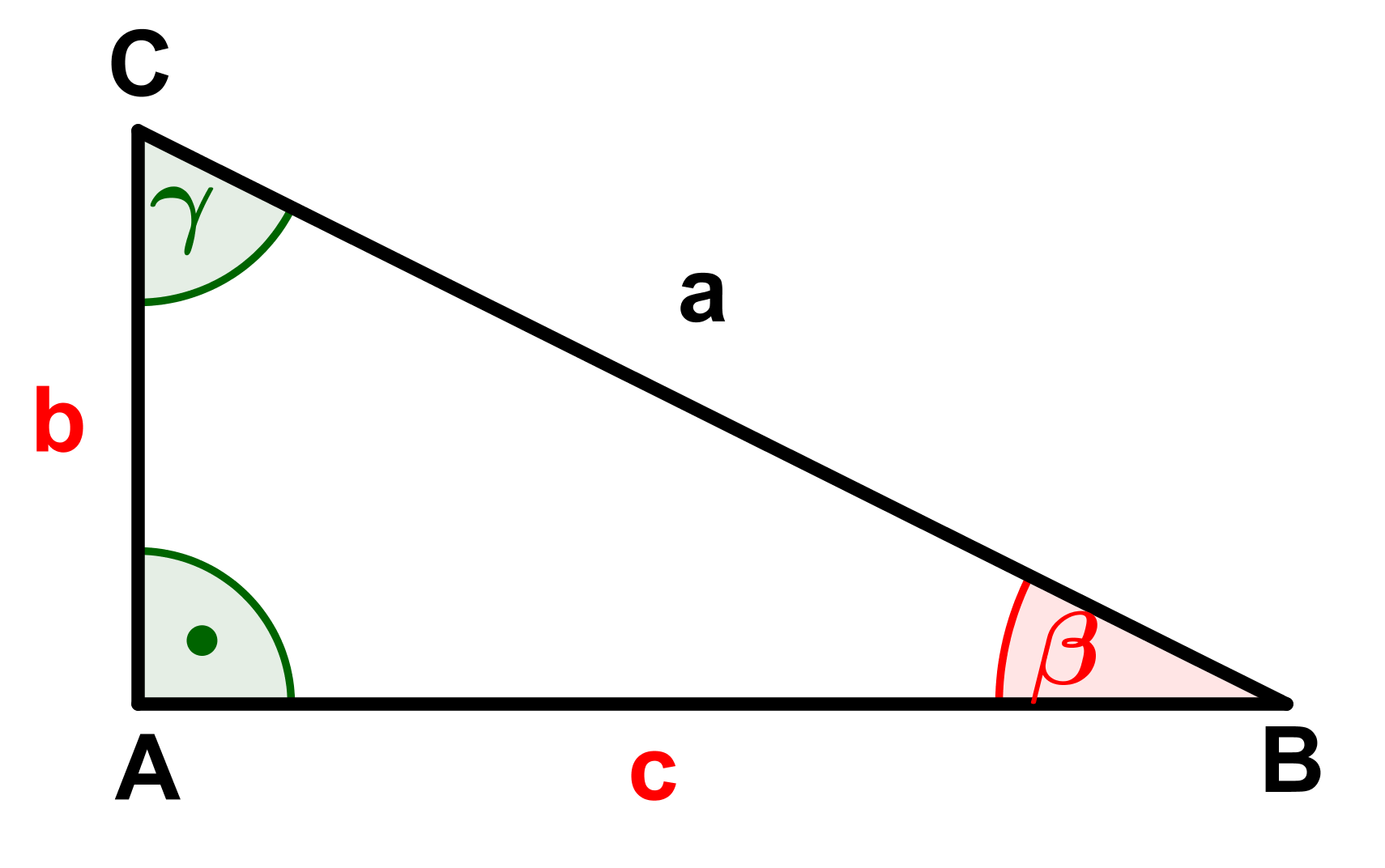
Calculate the missing sides and angles (marked red) of the triangles
- 2
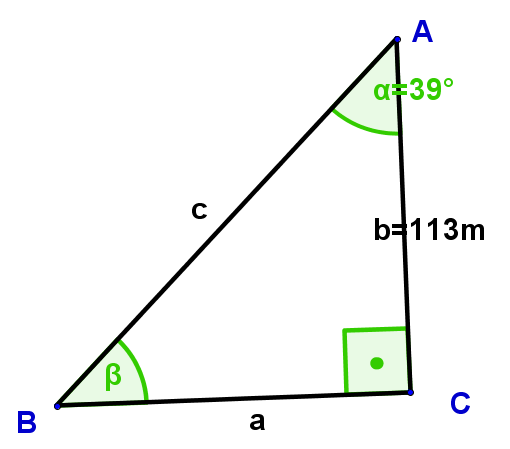
In a right triangle with and compute the length of .
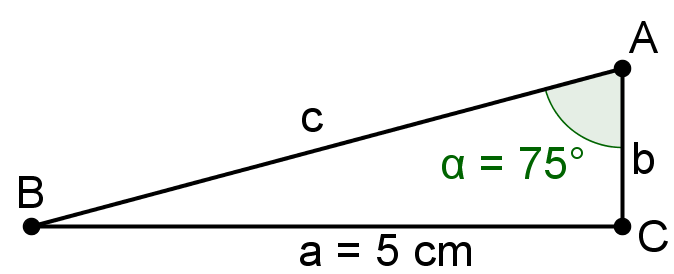
- 3
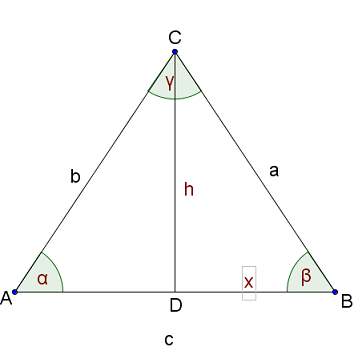
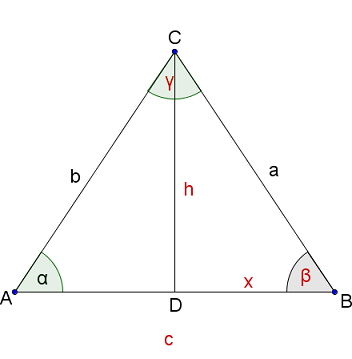
Calculate the missing sides and angles of the isosceles triangle with . Note that we are looking at general isosceles triangles that are not necessarily right-angled.
a=44.2cm
c=63.4cm
a=114.5m
=32.3°
c=35.4cm
=43.9°
h=14.8cm
28.3°
a=146.4m
h=58.4m
- 4
A triangle with a right angle at , with side has an angle . First make a sketch and then calculate all the missing sides and the other angle .
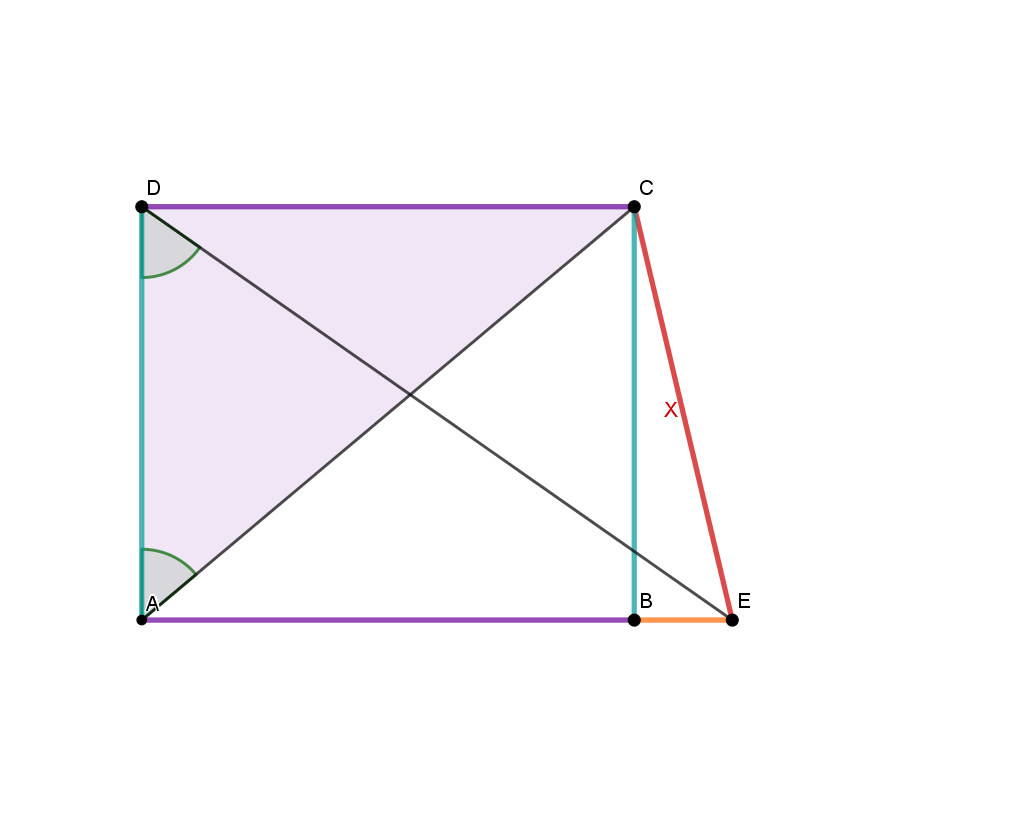
- 5
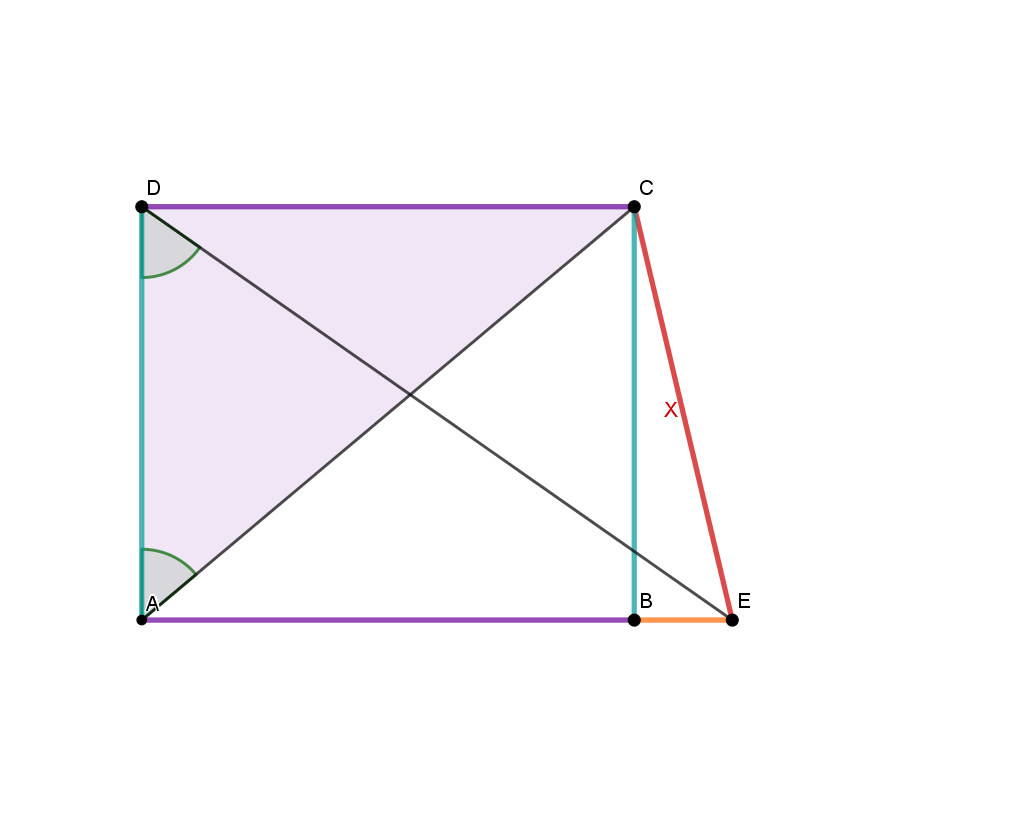
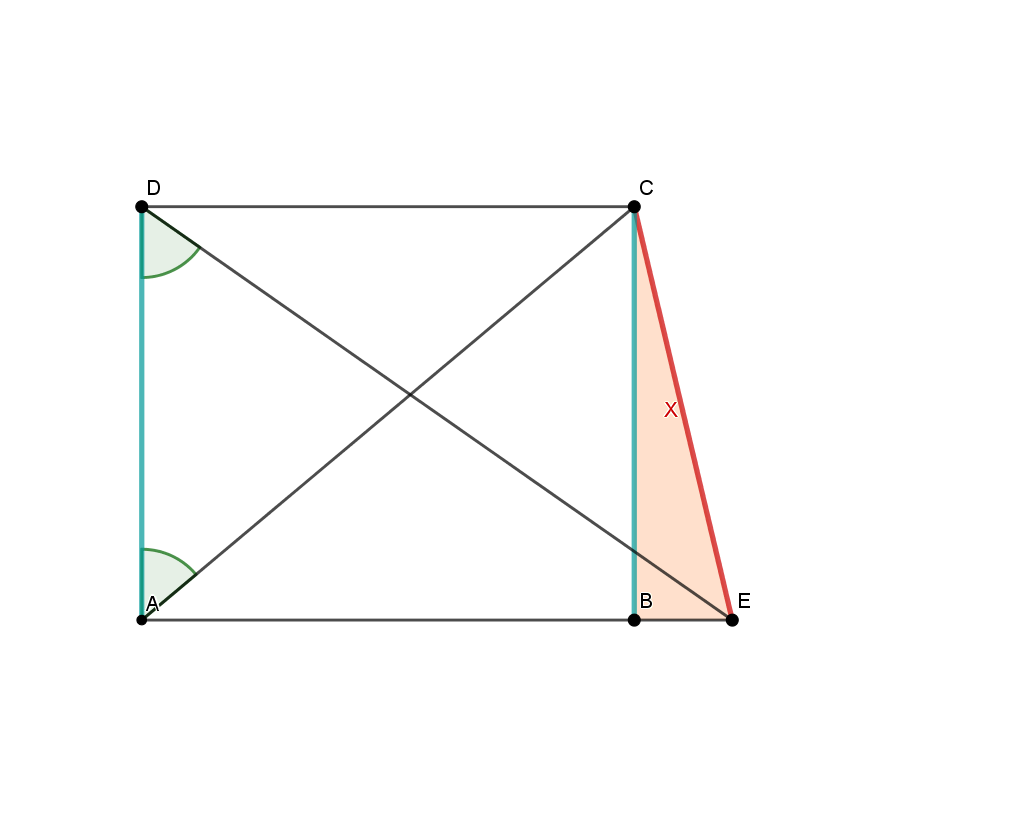
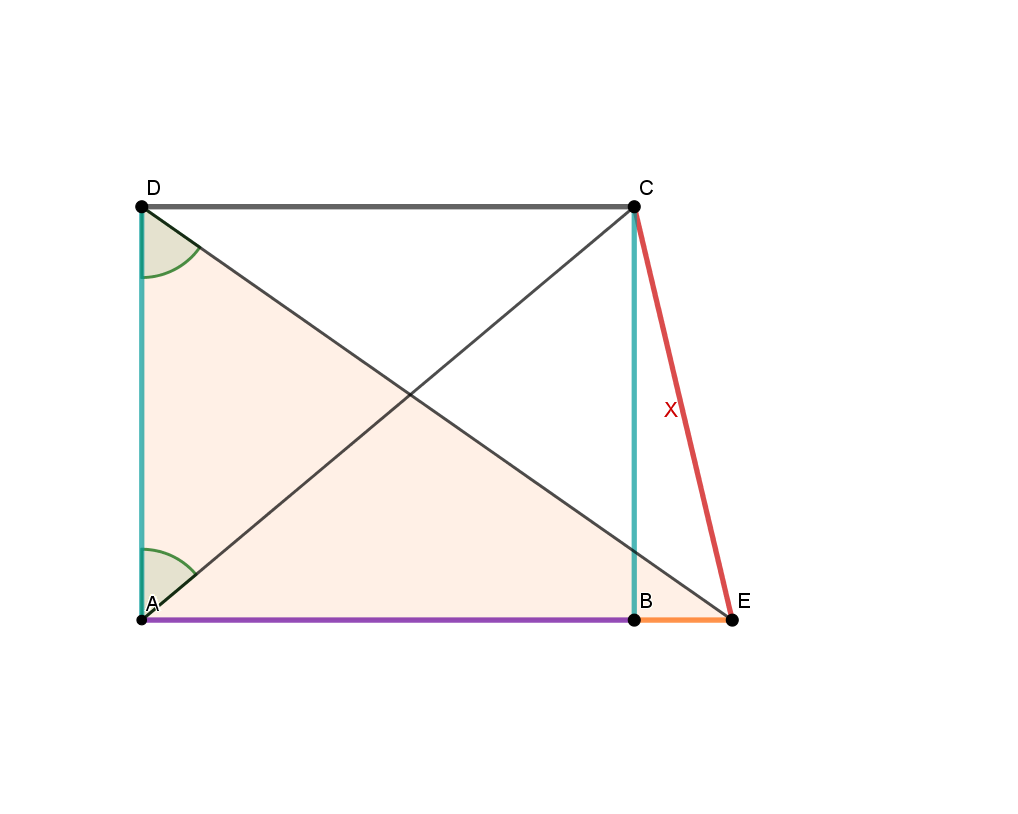
Sketch a rectangle with sides and and calculate the angles
between a diagonal and the sides
between both diagonals
- 6
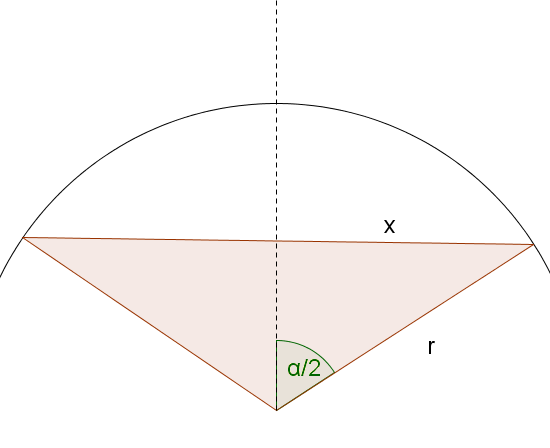
In a circle with radius the chord has the central angle .
What is the length of the chord?
- 7
Heike is tall. How long is her shadow when the sun's rays hit the ground at an angle of ? Give the result in metres rounded to 2 decimal places.
m - 8
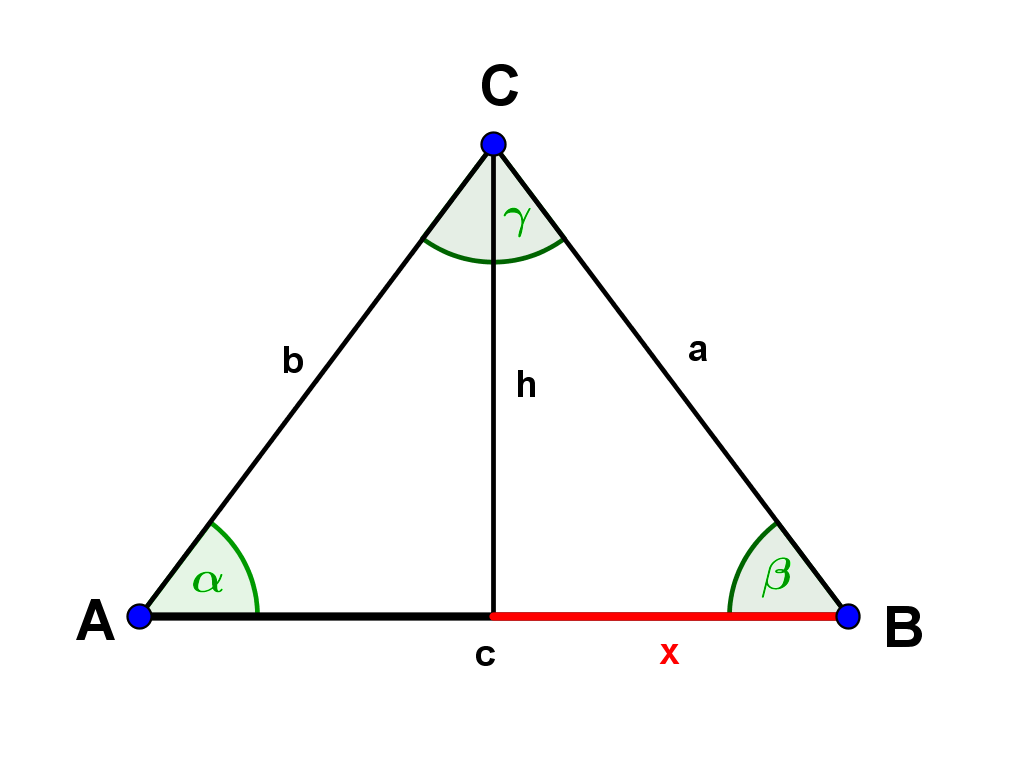
Calculate the missing sides and angles of the isosceles triangle with .
a = 44.2cm
c = 63.4cm
a = 114.5m
= 32.3°
c = 35.4cm
= 43.9°
= 14.8cm
= 28.3°
a = 146.4m
= 58.4m
- 9
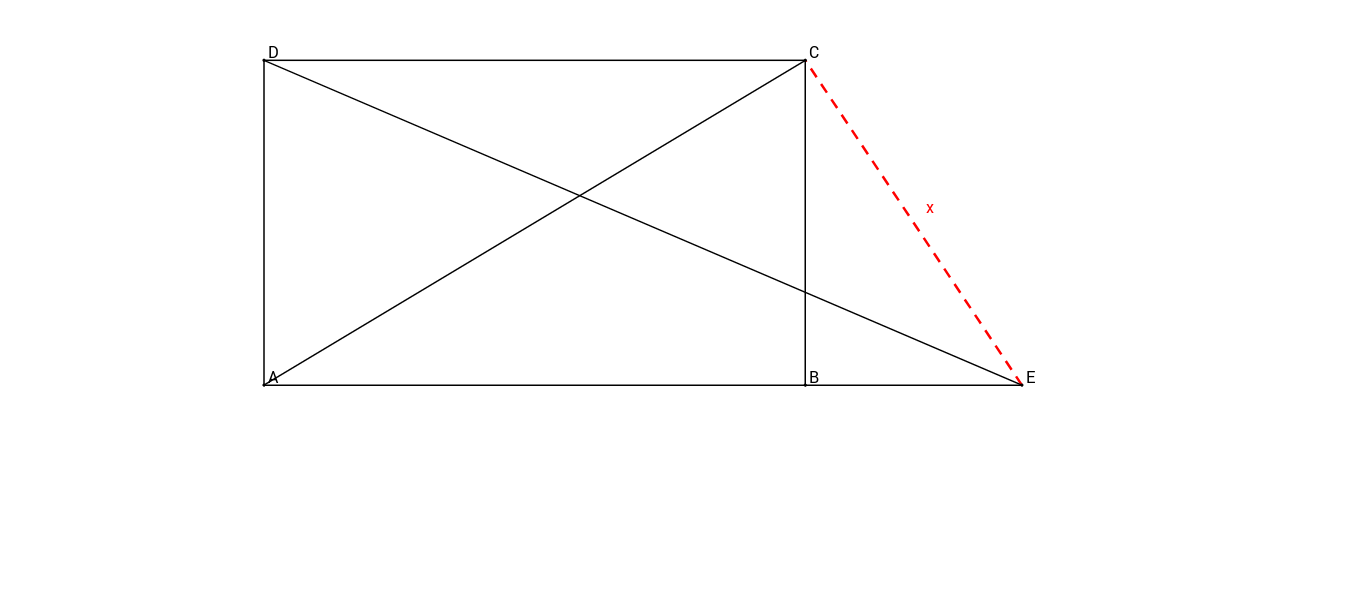
This sketch, which is not to scale, shows a trapezoid with the lengths:
Calculate the distance of
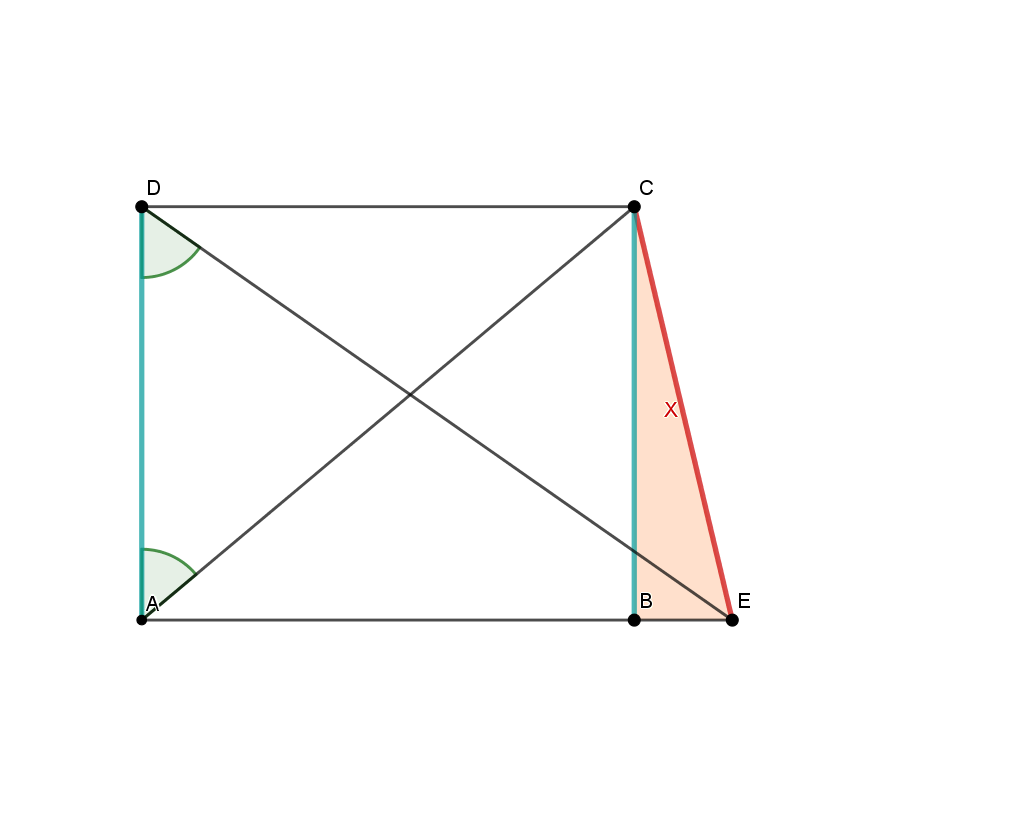
- 10
This sketch, which is not to scale, shows a kite quadrilateral with symmetry axis and the sides: , ,
Compute the angles and .
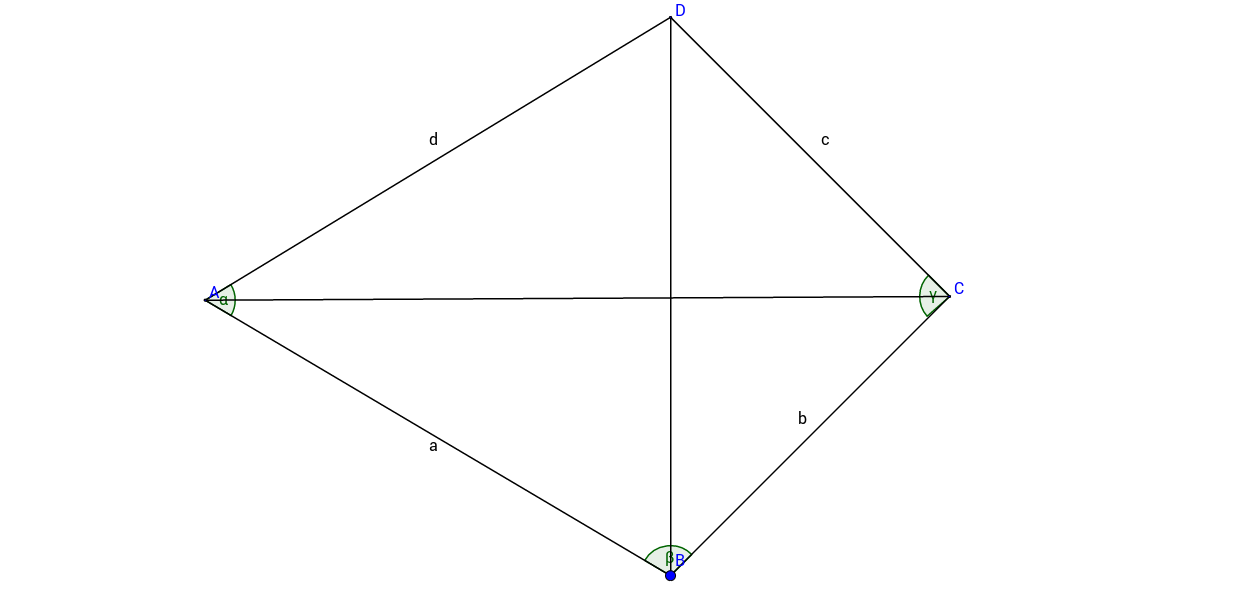
- 11
This sketch, which is not to scale, shows a rectangle with side lengths and .
Calculate the angle .
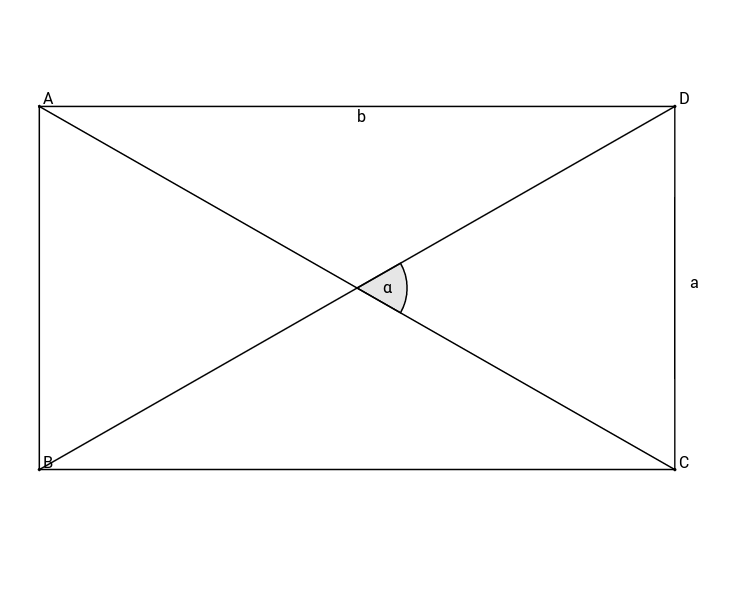
- 12
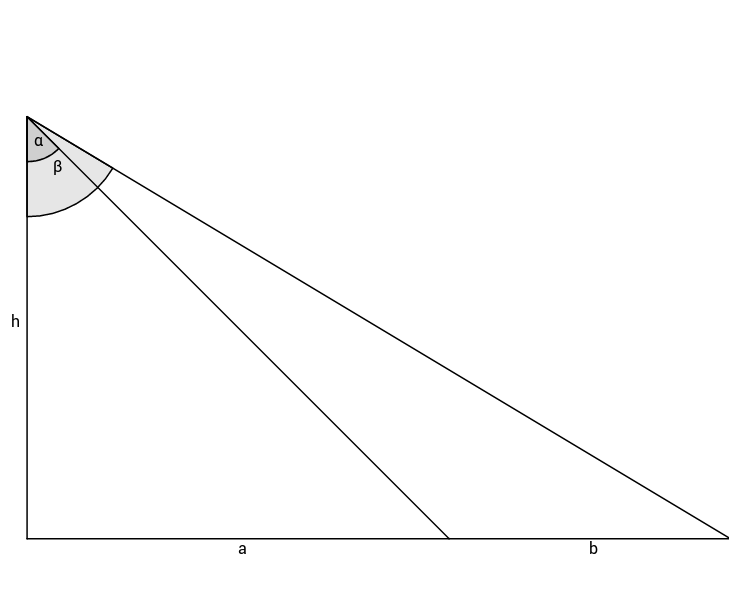
This sketch shows a right triangle, not true to size, with height and angles and .
Calculate the side lengths and .