Exercises: systems with two variables
Solve the following systems of equations with 2 equations and 2 variables first graphically and then by computation.
For this task you need the following basic knowledge: System of linear equations
Linear system with two variables
A linear system of equations is composed of several linear equations with common unknowns (variables), all of which are to be satisfied.
Graphical solution
To solve the system of equations graphically, you can resolve the individual lines for and draw the corresponding lines into a coordinate system. Then you only need to read off the coordinates of the intersection of the two lines.

The intersection point is at and . Thus the solution set is .
Solution by computation
In this case, the elimination by equating coefficients is a good choice, since you have already solved both equations for in order to solve them graphically.
Set and equal and solve for .
Plug the obtained value for into one of the equations, e.g. .
Now, you can write down the solution set.
For this task you need the following basic knowledge: System of linear equations
Linear system with two variables
A linear system of equations is composed of several linear equations with common unknowns (variables), all of which are to be satisfied.
Graphical solution
To solve the system of equations graphically, you can resolve the individual lines for and draw the corresponding lines into a coordinate system. Then you only need to read off the coordinates of the intersection of the two lines.

The intersection is at and . Thus the solution set is .
Solution by computation
In this case, the elimination by equating coefficients is a good choice, since you have already solved both equations for in order to solve them graphically.
Set and equal and solve for .
Plug the obtained value for into one of the equations, e.g. .
Now, you can write down the solution set.
For this task you need the following basic knowledge: System of linear equations
Linear system with two variables
A linear system of equations is composed of several linear equations with common unknowns (variables), all of which are to be satisfied.
Graphical solution
To solve the system of equations graphically, you can resolve the individual lines for and draw the corresponding lines into a coordinate system. Then you only need to read off the coordinates of the intersection of the two lines.
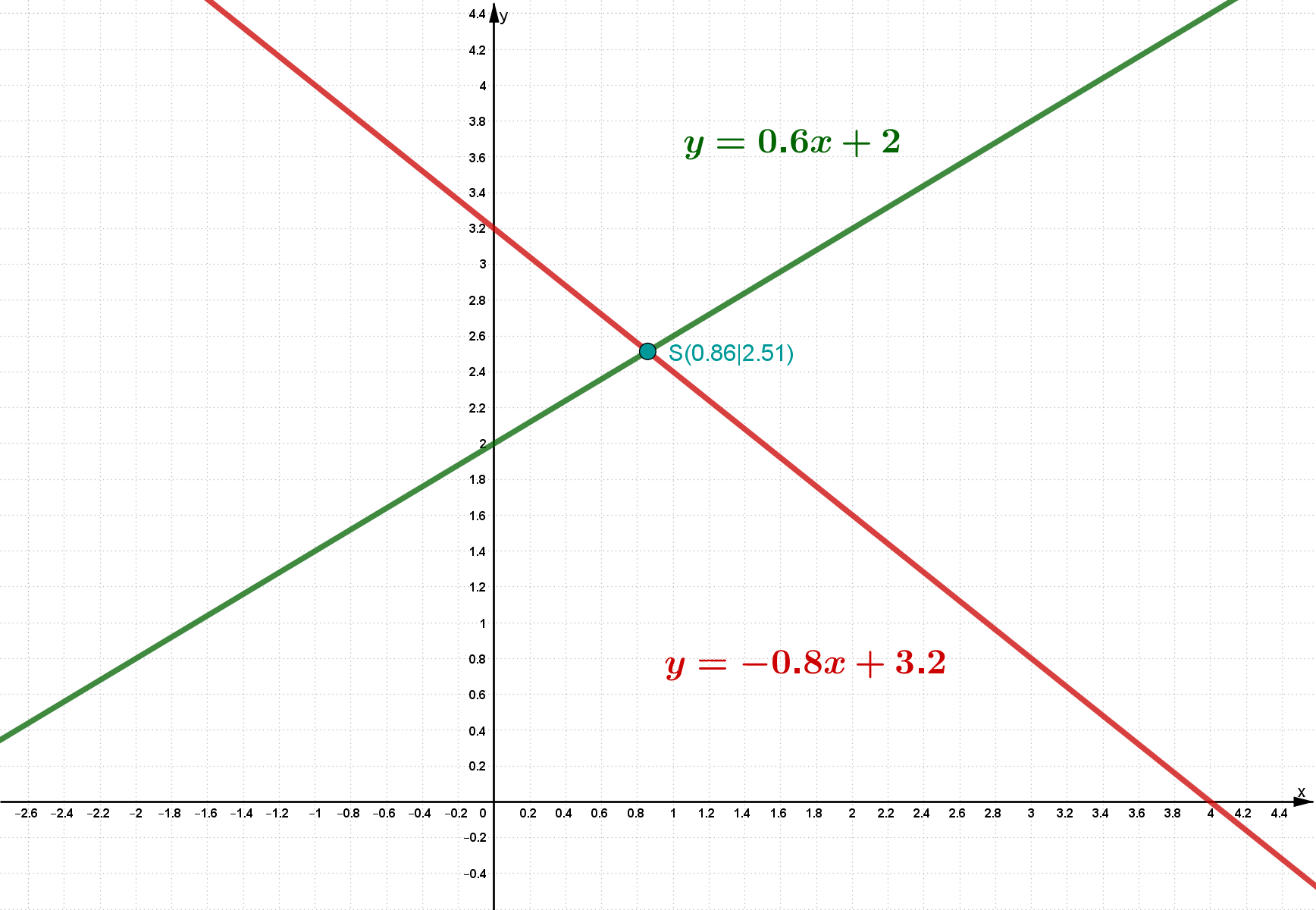
The intersection is at and . Thus the solution set is .
Solution by computation
In this case, the elimination by equating coefficients is a good choice, since you have already solved both equations for in order to solve them graphically.
Set and equal and solve for .
Plug the obtained value for into one of the equations, e.g. .
Now, you can write down the solution set.