Exercises: Distances, parallel and perpendicular lines
- 1
Determine the equation of the line that passes through the point and is perpendicular to the given line .
For this task you need the following basic knowledge: Slope/Gradient of a line
First determine the slope of the line perpendicular to , where being perpendicular implies
Plug in the known values.
So the line we are looking for has a slope of .
Now determine the -axis intercept of the line through by substituting the point into the general line equation.
Plug in the values.
Simplify and add .
So the line equation is .
For this task you need the following basic knowledge: Slope/Gradient of a line
First determine the slope of the line perpendicular to , where being perpendicular implies
Plug in the known values.
So the line we are looking for has a slope of .
Now determine the -axis intercept of the line through by substituting the point into the general line equation.
Plug in the values.
Simplify and add 2.
So the line equation is .
For this task you need the following basic knowledge: Slope/Gradient of a line
First determine the slope of the line perpendicular to , where being perpendicular implies
Plug in the known values.
So the line we are looking for has a slope of .
Now determine the -axis intercept of the line through by substituting the point into the general line equation.
Plug in the values.
Simplify and add 2.
So the line equation is .
For this task you need the following basic knowledge: Slope/Gradient of a line
First determine the slope of the line perpendicular to , where being perpendicular implies
Plug in the known values.
So the line we are looking for has a slope of .
Now determine the -axis intercept of the line through by substituting the point into the general line equation.
Plug in the values.
Simplify and add .
So the line equation is .
For this task you need the following basic knowledge: Slope/Gradient of a line
First determine the slope of the line perpendicular to , where being perpendicular implies
Plug in the known values.
So the line we are looking for has a slope of .
Now determine the -axis intercept of the line through by substituting the point into the general line equation.
Plug in the values.
Simplify and subtract 6.
So the line equation is .
For this task you need the following basic knowledge: Slope/Gradient of a line
First determine the slope of the line perpendicular to , where being perpendicular implies
Plug in the known values.
So the line we are looking for has a slope of .
Now determine the -axis intercept of the line through by substituting the point into the general line equation.
Plug in the values.
Simplify and add 6.
So the line equation is .
- 2
Determine the equation of the line that is parallel to the line and passes through the point .
: ;
For this task you need the following basic knowledge: Slope/Gradient of a line
;
For the line to be parallel to , it must have the same slope.
The slope of a line is the variable of the general line equation.
Setting up the line equation
Plug and into the the general line equation.
↓ solve for
Plug and into the general line equation.
Line equation:
: ;
For this task you need the following basic knowledge: Slope/Gradient of a line
;
For the line to be parallel to , it must have the same slope.
The slope of a line is the variable of the general line equation.
Setting up the line equation
Plug and into the the general line equation.
↓ solve for
Plug and into the general line equation.
Line equation:
: ;
For this task you need the following basic knowledge: Slope/Gradient of a line
;
For the line to be parallel to , it must have the same slope.
The slope of a line is the variable of the general line equation.
Setting up the line equation
Plug and into the the general line equation.
↓ solve for
Plug and into the general line equation.
Line equation:
: ;
For this task you need the following basic knowledge: Slope/Gradient of a line
;
For the line to be parallel to , it must have the same slope.
The slope of a line is the variable of the general line equation.
Setting up the line equation
Plug and into the the general line equation.
↓ solve for
Plug and into the general line equation.
Line equation:
- 3
Determine the equation of the straight line through ...
the point and being parallel to the -axis.
For this task you need the following basic knowledge: Line equation
Parallel to the -axis, means that the slope is .
Plug and into the general line equation.
Assemble to a line equation.
the point and is parallel to the bisector of the 2nd quadrant (the diagonal pointing down).
For this task you need the following basic knowledge: Line equation
Parallel to the bisector of the 2nd quadrant means the line has the same slope as the bisector.
The slope of the bisector of the 2nd quadrant is -1
Plug into the straight line equation in order to calculate .
Plug and into the general equation of a straight line.
the point and is parallel to the -axis.
For this task you need the following basic knowledge: Line equation
Parallel to the -axis means that there is no function equation, since an -value is assigned an infinite number of -values.
The straight line can therefore only be described as the -value of .
the point and is parallel to the bisector of the 1st quadrant (the diagonal pointing up).
For this task you need the following basic knowledge: Line equation
The line being parallel to the bisector of the 1st quadrant means it has the same slope.
The slope of the bisector of the 1st quadrant is .
Substitute and into the general line equation and solve for .
Substitute and into the general line equation.
the origin and is parallel to the straight line with and .
For this task you need the following basic knowledge: Line equation
Passing through the origin means, the -axis intercept is .
Being parallel to the straight line means that the line has the same slope as .
Calculate the slope using the difference quotient .
Substitute and into the general line equation.
- 4
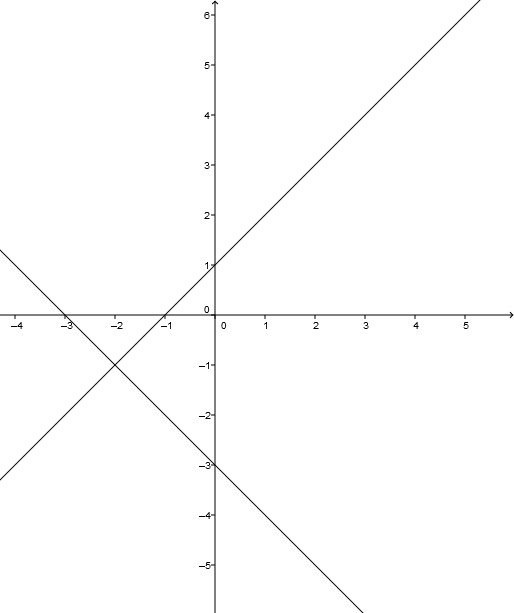
Two perpendicular lines intersect at .
Determine at least one possible line equations.
For this task you need the following basic knowledge: Linear function
We need to construct two perpendicular lines with the point of intersection . As you can already see from the problem setting, there are several ways to choose two such straight lines. One possibility is given below. A good criterion to check whether the two chosen straight lines are perpendicular to each other is to check whether .
For instance, choose the line with and with . Then and we have and . So the point of intersection is on the two lines and they are perpendicular to each other.
Caution:
You may also choose the line , which is parallel to the -axis and passes through the point . Then there is exactly one line perpendicular to it and passing through this point, namely that one given by and running parallel to the - axis. However, does not describe a function but a relation!
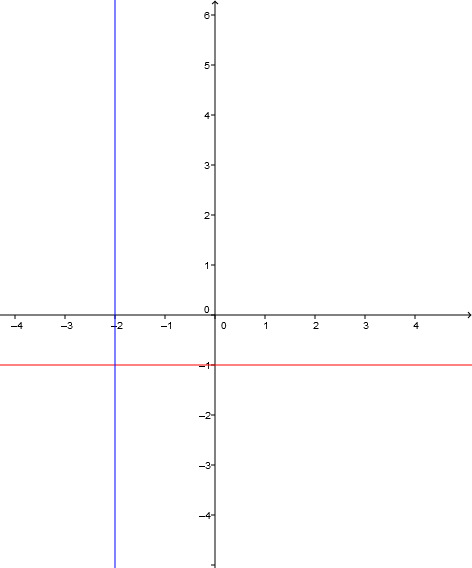
- 5
Calculate the distance of the line to the origin.
For this task you need the following basic knowledge: Slope/Gradient of a line
The shortest connection of any point on the line to the origin is a second line which passes through the origin and is perpendicular to .
Passing through the origin means .
Being perpendicular means
Plug in the known value of .
So the perpendicular line has the line equation .
Now calculate the intersection point of the two lines by setting equal their line equations.
↓ Plug in the line equations.
↓ Get the variable on the left side
Now substitute into one of the line equations to determine .
Plug in .
The intersection of the lines and is therefore at .
Now determine the distance of the origin to the calculated intersection point , this is exactly the shortest distance of the line to the origin.
Plug in the values.
Simplify.
The shortest distance of the line to the origin is therefore .
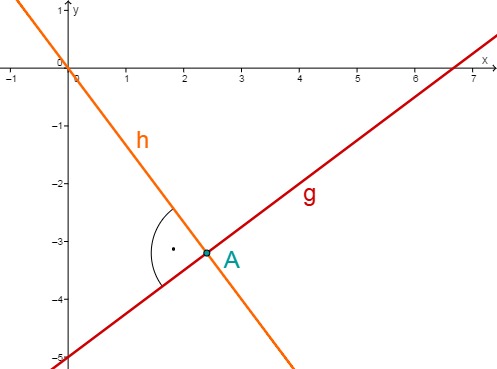
For this task you need the following basic knowledge: Slope/Gradient of a line
The shortest connection of any point on the line to the origin is a second line which passes through the origin and is perpendicular to .
Passing through the origin means .
Being perpendicular means
Plug in the known value of .
So the perpendicular line has the line equation .
Now calculate the intersection point of the two lines by setting equal their line equations.
↓ Plug in the line equations.
↓ Get the variable on the left side
↓ Get the 2 to the right side.
Now substitute into one of the line equations to determine .
Plug in .
The intersection of the lines and is therefore at .
Now determine the distance of the origin to the calculated intersection point , this is exactly the shortest distance of the line to the origin.
Plug in the values.
Simplify.
The shortest distance of the line to the origin is therefore approximately .

- 6
Calculate the distance of the parallel lines g: and h: .
Distance between two parallel lines
The shortest path between two parallel lines can be read off along a normal (=perpendicular line) of the two parallel lines .
The distance of the straight lines is equal to the distance between the two intersection points of the normal line with the parallel lines .
Sketch
The construction looks as follows:
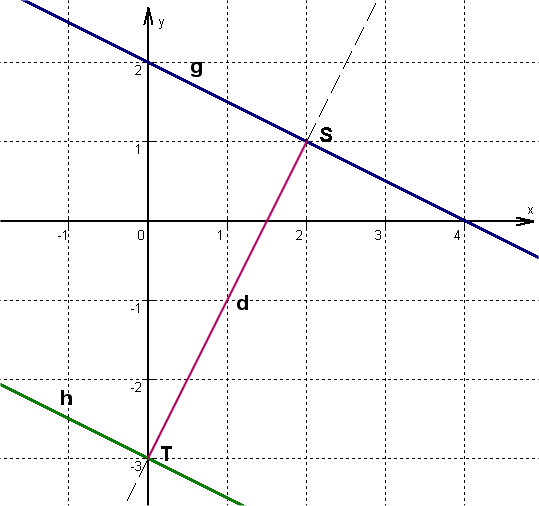
↓ Plug in the slope
↓ Simplify the right-hand side
Now set up the line equation.
For this, you need a point , which lies on line h. For instance, you may take the -axis intercept of , which is . But any other point is also correct.
Plug and into the general line equation for d to get the parameter .
↓ multiply out
↓ Plug and into the general line equation.
Determine the intersection of d and g
First calculate the -coordinate of the intersection point .
Normal line d:
Paralle line g:
Equate both function equations:
Now calculate the -coordinate of the intersection point .
↓ Plug in x = 2 .
↓ compute
Hence the point has the following coordinates:
Determine the distance between points S and T.
Determine the distance in -direction.
,
Calculate the difference of the -values of and .
Determine the distance in -direction.
,
Calculate the difference of the -values.
Determine the distance in a direct line between the points.
This is done by the Pythagoraen Theorem:
↓ compute both squares
↓ add
↓ take the root
If you enter into a calculator and round the result to two decimal places, you get:
Result
The distance between the two lines is .
- 7
Consider the equation .
Zeichne die Gerade zu der Gleichung in ein Koordinatensystem.
For this task you need the following basic knowledge: Lines in coordinate systems
Choose any point on the line, e.g. the -axis-intercept , and go two to the right and three upwards, which corresponds to a slope of . Connect the two points to obtain a straight line.
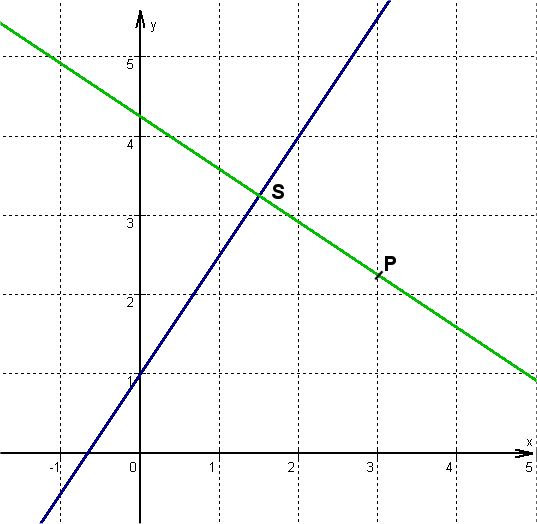
Set up the equation of the perpendicular line through the point .
For this task you need the following basic knowledge: Slope/Gradient of a line
You obtain the slope of the perpendicular (=normal) line by dividing -1 by the original slope.
↓ Plug the coordinates of and into the general line equation.
↓ Solve for .
Substitute and into the general line equation.
Draw the line in the same coordinate system as the line from exercise 1.
For this task you need the following basic knowledge: Slope/Gradient of a line
Choose any point on the line, e.g. the given point and go, according to the gradient , units to the left and units upwards. Connect the two points to obtain a straight line. (see sketch in subtask 1)
Calculate the intersection of the two lines.
Calculating the -coordinate
Set the straight line functions equal to obtain the point of intersection.
↓ Get to one side.
↓ Factor out and add the fractions.
Calculating the -coordinate
Plug into one of the line equations, e.g. that of the given line.
S